Hypothesis Testing Example - BMS Diabetes Research

BMS Diabetes Research

Title: Co-stimulation modulation with abatacept in patients with recent-onset type 1 diabetes. A randomized, double-blind, placebo-controlled trial.

Objective: The immunopathogenesis of type 1 diabetes mellitus is associated with T-cell autoimmunity. To be fully active, immune T cells need a co-stimulatory signal in addition to the main antigen-driven signal. Abatacept modulates co-stimulation and prevents full T-cell activation. We evaluated the effect of abatacept in recent-onset type 1 diabetes.

Year: 2011

Source: The Lancet

Link: http://www.sciencedirect.com/science/article/pii/S0140673611608866

Clinical Area: Cardiology

Sample Size Section in Paper/Protocol:

“Among baseline subjects, the mean log(c-peptide + 1) values are 0.248 with RMSE =0.179. The corresponding geometric-like mean C-peptide value is 0.282 pmol/mL obtained using the inverse transformation exp(0.248) – 1. Using standard equations it was determined that a sample size of 108 subjects randomized to active treatment versus placebo in a 2:1 ratio would provide power of 85% to detect a 50% increase in the geometric-like mean C-peptide with the active treatment related to the placebo group using a test at the 0.05 level (one-sided), with 10% loss to follow-up. Interim analysis for endpoint treatment effect was done and reported to the data and safety monitoring board once in accordance to the method of Lan and DeMets with O'Brien-Fleming boundaries.”

Summary of Necessary Parameter Estimates for Sample Size Calculation:

Parameter	Value
Significance Level	0.05
Expected Placebo Mean	0.248
Expected Treatment Mean	0.353
Common Standard Deviation (RMSE)	0.179
Power	85%
Number of Interim Analyses	1
Dropout	10%

Step 1:
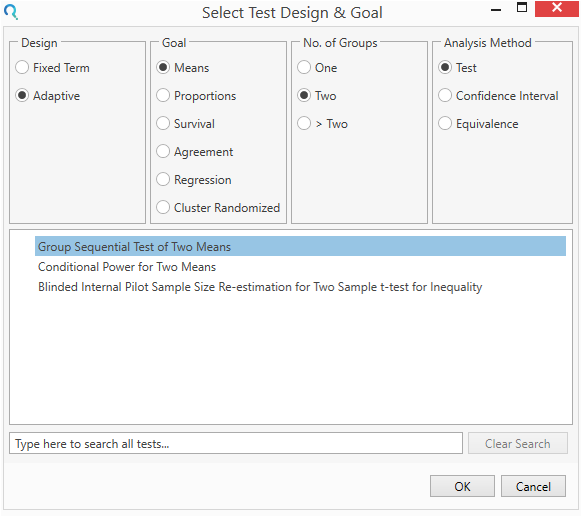
Select the Group Sequential Test of Two Mean Table from the Select Test Design & Goal window.

This can be done using the radio buttons or alternatively, you can use the search bar at the end of the Select Test Design & Goal window.

Step 2:
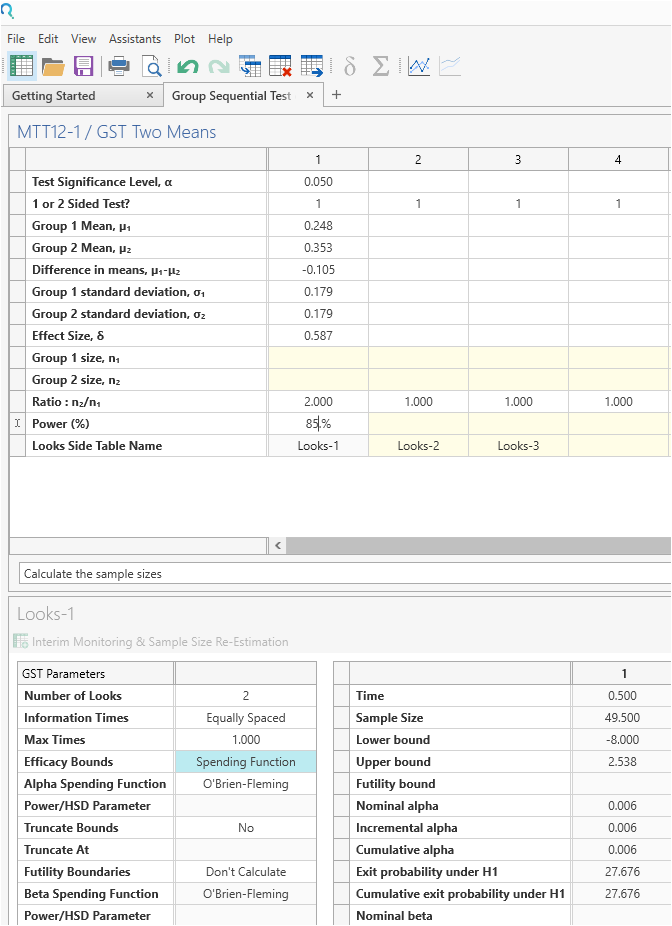
Enter the values for sample size calculation taken from the study design statement.

Setting the “Number of looks” row to 2 specifies one interim analysis and one endpoint analysis.

The O’Brien Fleming boundaries will be selected by default. No futility analysis was included in this study.

Set the standard deviation in both groups to the same value to indicate a common variances solution.

Step 3:
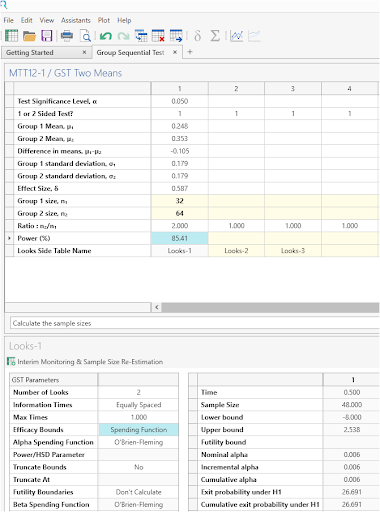
Click Run to solve for sample size

The analysis gives a sample size of 32 and 64 giving 82.4% power.

The sample sizes adjusted for dropout were 36 and 72 (sample sizes divided by 0.9).

This gives the total sample size of 108 given in the study design.

Step 4:
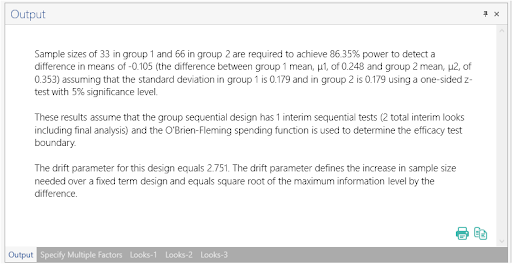
Once the calculation is completed, nQuery Advanced provides an output statement summarizing the results. It States:

Output Statement:

Sample sizes of 32 in group 1 and 64 in group 2 are required to achieve 85.41% power to detect a difference in means of -0.105 (the difference between group 1 mean, μ1, of 0.248 and group 2 mean, μ2, of 0.353) assuming that the standard deviation in group 1 is 0.179 and in group 2 is 0.179 using a one-sided z-test with 5% significance level. These results assume that the group sequential design has 1 interim sequential tests (2 total interim looks including final analysis) and the O'Brien-Fleming spending function is used to determine the efficacy test boundary.

The drift parameter for this design equals 2.709. The drift parameter defines the increase in sample size needed over a fixed term design and equals square root of the maximum information level by the difference.

Step 5:
nQuery Advanced also provides graphs and the details of the interim analysis.

Sample Size Determination Example

AREA: Means | Cardiology

BMS Diabetes Research

Title: Co-stimulation modulation with abatacept in patients with recent-onset type 1 diabetes. A randomized, double-blind, placebo-controlled trial.

Email me this example in PDF format

Email me this example in PDF format

Free 14 Day Trial

LOCATION